Knitted Hyperbolic Surfaces
Large pieces of high-curvature hyperbolic space are better crocheted than
knitted, I think, unless you are willing to use double-pointed needles (which I
am not) or have access to a lot of long interchangeable needle cables. Still, I've made some pieces of hyperbolic surfaces.
It's fun to toss these at students and ask (a) is the curvature positive or
negative on these objects? and (b) which one has greater curvature?


I had knitted the above bits from the inside out, so to speak, by beginning
with a small number of stitches and putting an increase on every stitch or
every-other stitch. (That's exactly what one does when crocheting.)
The outside-in recipe is as follows:
Cast on as many stitches as your needle will hold. (I use circular needles,
always.)
2:1 stitch ratio? *K2 togtbl* each row until you feel done. Cast off.
3:2 stitich ratio? *K1, K2togtbl* each row until you feel done. Cast off.
4:3 stitch ratio? *K2, K2togtbl* each row until you feel done. Cast off.
Taken together, Ari and I agreed they look much like Xmas ornaments. 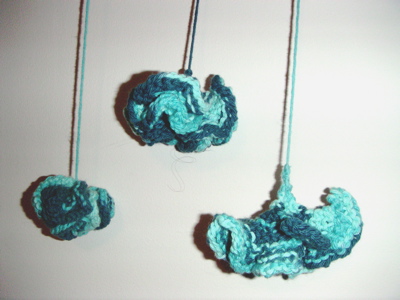
A few items pictured publicly only on 
A nice, loose pseudosphere may be
made in the following way:
Cast on as many stitches as your circular needle will hold. Join without
twisting to work in the round.
*K10, K2togtbl* until one stitch remains. Yes, really, that works, and yes,
really, that's all there is to it.
One cool thing that can be done with a hyperbolic octagon is folding it into
pants. Knitting instructions for baby pants can be found in Making Mathematics with Needlework and a
customizable version of the pattern is at the Wolfram
Demonstrations Project.
I made an eight-colored pair of hyperbolic pants; really, they are a dual map to K8 embedded on
a 2-holed torus.
I did once make a hyperbolic
toddler tutu...






